Answer:
1) The value of
 is 12.
is 12.
2) The length of the diagonal is 49.
Explanation:
1) If the glass is a rectangle, then both diagonal must have the same length, that is, the following equation must be solved:
 (1)
(1)
We need some algebraic handling to determine the value of
 :
:

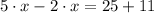
![[5+(-2)] \cdot x = 36](https://img.qammunity.org/2022/formulas/mathematics/high-school/symm88awp159jbbrqf5chlwf8ly61lhdq1.png)
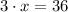
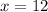
The value of
 is 12.
is 12.
2) If we know that
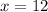 , then the length of the diagonal (
, then the length of the diagonal (
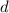 ) is:
) is:
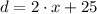
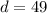
The length of the diagonal is 49.