Question

How do I write the equation of the parabola in intercept form​

Answer 1

The equation of the parabola in intercept form is
`y = -(3)/(4)\cdot x\cdot (x-4)`.

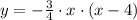Explanation:

The equation of the parabola in intercept form is defined by following formula:

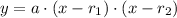`y = a\cdot (x-r_(1))\cdot (x-r_(2))` (1)

Where:

`x` - Independent variable.

`y` - Dependent variable.

`a` - Vertex constant.

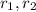`r_(1), r_(2)` - Intercepts of the parabola.

According to the graph, we find that intercepts are 0 and 4, respectively, and a point in the parabola is
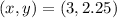`(x,y) = (3, 2.25)`. If we know that
`r_(1) = 0`,
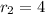`r_(2) = 4` and
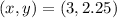`(x,y) = (3, 2.25)`, then the vertex constant is:

`a = (y)/((x-r_(1))\cdot (x-r_(2)))`

`a = -(3)/(4)`

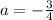
The equation of the parabola in intercept form is
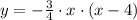`y = -(3)/(4)\cdot x\cdot (x-4)`.