Question

If


`\binom{13}{7}`
N has a half-life of about 10.0 min, how long will it take for 20 g of the isotope to decay to 1.9 g? [2 marks] 2. Radon-22​

Answer 1

the time it will take the element to decay to 1.9 g is 34.8 mins.

Step-by-step explanation:

Given;

half life of Nitrogen, t = 10 min

initial mass of the element, N₀ = 20 g

final mass of the element, N = 1.9 g

The time taken for the element to decay to final mass is calculated as follows;

time (min) mass remaining

0 ----------------------------------20 g

10 mins ------------------------- 10 g

20 mins ------------------------- 5 g

30 mins -------------------------- 2.5 g

40 mins --------------------------- 1.25 g

Interpolate between 2.5 g and 1.25 to obtain the time for 1.9 g

30 min ------------------------- 2.5 g

x ----------------------------------- 1.9 g

40 min -------------------------- 1.25 g

`(30 - x)/(40- 30) = (2.5 - 1.9)/(1.25 - 2.5) \\\\-1.25(30-x) = 6\\\\-37.5 + 1.25x = 6\\\\1.25x = 6+37.5\\\\1.25x = 43.5\\\\x = (43.5)/(1.25) \\\\x = 34.8 \ mins`

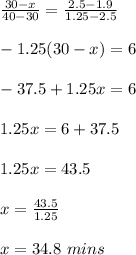
Therefore, the time it will take the element to decay to 1.9 g is 34.8 mins.