Answer:
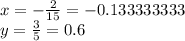
Explanation:
−12x+9y=7
3x+4y=2
In order to solve by elimination, coefficients of one of the variables must be the same in both equations so that the variable will cancel out when one equation is subtracted from the other.
−12x+9y=7,3x+4y=2
To make −12x and 3x equal, multiply all terms on each side of the first equation by 3 and all terms on each side of the second by −12.
3(−12)x+3×9y=3×7,−12×3x−12×4y=−12×2
Simplify.
−36x+27y=21,−36x−48y=−24
Subtract −36x−48y=−24 from −36x+27y=21 by subtracting like terms on each side of the equal sign.
−36x+36x+27y+48y=21+24
Add −36x to 36x. Terms −36x and 36x cancel out, leaving an equation with only one variable that can be solved.
27y+48y=21+24
Add 27y to 48y.
75y=21+24
Add 21 to 24.
75y=45
Divide both sides by 75.
y=3/5
Substitute 3/5 for y in 3x+4y=2. Because the resulting equation contains only one variable, you can solve for x directly.
3x+4×(3/5)=2
Multiply 4 times 3/5
3x+12/5=2
Subtract 12/5 from both sides of the equation.
3x=-2/5
Divide both sides by 3.
x=-2/15
The system is now solved.
x=−2/15 ,y=3/5