Question

Testing:

H0:μ=54.5

H1:μ<54.5

Your sample consists of 48 subjects, with a mean of 54.1 and standard deviation of 1.8.

Calculate the test statistic, rounded to 2 decimal places.

t=

Answer 1

t = -1.54.

Explanation:

The test statistic is:

`t = (X - \mu)/((\sigma)/(√(n)))`

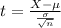
In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

H0:μ=54.5

This means that
`\mu = 54.5`

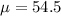
Your sample consists of 48 subjects, with a mean of 54.1 and standard deviation of 1.8.

This means, respectively, that
`n = 48, \mu = 54.1, \sigma = 1.8`

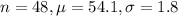
Test statistic

`t = (X - \mu)/((\sigma)/(√(n)))`

`t = (54.1 - 54.5)/((1.8)/(√(48)))`

`t = -1.54`

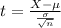
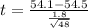
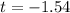
The test statistic is t = -1.54.