Answer:
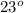 and
and
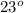
Explanation:
An isosceles triangle has two of its sides and angles to be equal. Let each of the unknown equal angles be represented by x. Since one of its angles measures 134°, then;
x + x + 134° =
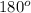
2x + 134° =
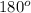
2x =
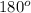 - 134°
- 134°
= 46
x =
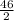
=
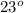
x =
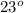
The other angles that could be in the isosceles triangle are
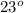 and
and
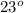 .
.