Question

A square pyramid has a base length of 4 inches. The height of each triangular face is 12 inches. What is the surface area of the pyramid?

Answer 1

The surface area of the given pyramid is 112
`inches^(2)`.

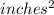Explanation:

Base length of the pyramid = 4 inches

Area of the base =
`length^(2)`

=
`4^(2)`

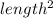
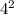
= 16

Area of its base = 16
`inches^(2)`

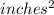
Area of one of its triangular surface =
`(1)/(2)` x base x height

=
`(1)/(2)` x 4 x 12

= 2 x 12

= 24
`inches^(2)`

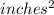
Area of all its four triangular surfaces = 4 x 24

= 96
`inches^(2)`

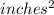
surface area of the pyramid = sum of areas of all its surfaces

= 16 + 96

= 112
`inches^(2)`

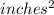
The surface area of the given pyramid is 112
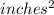`inches^(2)`.