Answer:
The Margin of Error in percentage is 5.88%
Explanation:
The values of the factors in the question are;
The mean increase in price per share, = $1.95
The number of companies in the sample, n = 100
The mean increase in price per share,
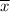 = $1.82
= $1.82
The standard deviation of the population, σ = $0.30
The margin of error, MOE, is given by the following formula;
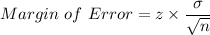
Where;
z = z-score, critical value = 1.96 at 95%
σ = The standard deviation of the population = $0.30
n = The sample size = 100
Therefore, we have;
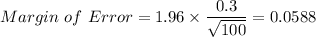
The Margin of Error in percentage = 0.0588 × 100 = 58.8%
The Margin of Error in percentage = 5.88%