Answer:
fem = -A β
Step-by-step explanation:
Faraday's law gives the induced electromotive source (emf)
fem =

the magnetic flux is
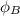 = B. A = B A cos θ
= B. A = B A cos θ
the bold are vectros. In this case the normal to the ring is parallel to the magnetic field so the angle is zero cos 0 = 1, also the area of the ring is constant
fem = -A
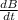
we carry out the derivative of the function B = α + β t
fem = -A β
so we see that there is an electromotive force in the ring.