The correct statement is option C.) The graph of function f is compressed horizontally by a scale factor of 1/4 to create the graph of function g.
When the expression
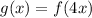 is used, it indicates a transformation of the original function
is used, it indicates a transformation of the original function
 . Specifically, the factor 4 inside the function parentheses affects the variable x. In this context, it implies a compression of the graph horizontally.
. Specifically, the factor 4 inside the function parentheses affects the variable x. In this context, it implies a compression of the graph horizontally.
To understand this, consider that for any given x-value in function g, the corresponding x-value in function f is 1/4 of that. As a result, the entire graph of function f is squished horizontally, making it narrower.
Options A and D suggest stretching, but the correct transformation is a compression due to the multiplication by 1/4. Option B suggests a vertical compression, which is not accurate in this context.
In summary, when
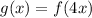 , the graph of function f is compressed horizontally by a scale factor of 1/4 to create the graph of function g.
, the graph of function f is compressed horizontally by a scale factor of 1/4 to create the graph of function g.