Given:
Principal value = $240,000
Rate of interest = 6% compounded continuously.
Time = 30 years
To find:
The amount after interest.
Solution:
The formula for amount after continuous compounding the interest is:
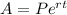
Where, P is principal, r is rate of interest and t is the number of years.
Putting
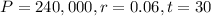 , we get
, we get
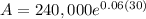

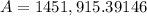
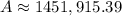
Therefore, Martins will pay $1451,915.39 for the house, including interest.