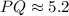Answer:
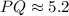
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
- Brackets
- Parenthesis
- Exponents
- Multiplication
- Division
- Addition
- Subtraction
Equality Properties
- Multiplication Property of Equality
- Division Property of Equality
- Addition Property of Equality
- Subtraction Property of Equality
Trigonometry
- [Right Triangles Only] SOHCAHTOA
- [Right Triangles Only] cosθ = adjacent over hypotenuse
Explanation:
Step 1: Identify
Angle θ = 55°
Adjacent Leg = PQ
Hypotenuse = 9
Step 2: Solve
- Substitute in variables [cosine]:

- [Multiplication Property of Equality] Isolate PQ:
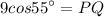
- Evaluate:
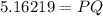
- Rewrite:
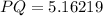
- Round: