Question

Terri Vogel, an amateur motorcycle racer, averages 129.01 seconds per 2.5 mile lap (in a 7 lap race) with a standard deviation of 2.26 seconds . The distribution of her race times is normally distributed. We are interested in one of her randomly selected laps. (Source: log book of Terri Vogel) Let X be the number of seconds for a randomly selected lap.

Required:
a. In words, define the random variable X.
b. X ~ ________(_____ , _____)
c. Find the percent of her laps that are completed in less than 130 seconds.
d. The fastest 3% of her laps are under
e. The middle 80% of her laps are from ___________seconds to ________seconds.

Answer 1

a. X is the time, in seconds, of a randomly selected lap of Vogel.

b. X~N(129.01,2.26)

c. 67% of her laps that are completed in less than 130 seconds.

d. So the answer is 124.8 seconds.

e. The middle 80% of her laps are from 126.1 seconds to 131.9 seconds.

Explanation:

Normal Probability Distribution:

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

Terri Vogel, an amateur motorcycle racer, averages 129.01 seconds per 2.5 mile lap (in a 7 lap race) with a standard deviation of 2.26 seconds

This means that
`\mu = 129.01, \sigma = 2.26`

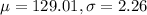
a. In words, define the random variable X.

The problem states that X is the time, in seconds, of a randomly selected lap of Vogel.

b. X ~ ________(_____ , _____)

Normal with mean and standard deviation. So

X~N(129.01,2.26)

c. Find the percent of her laps that are completed in less than 130 seconds.

The proportion is the pvalue of Z when X = 130. So

`Z = (X - \mu)/(\sigma)`

`Z = (130 - 129.01)/(2.26)`

`Z = 0.44`

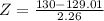
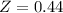
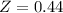`Z = 0.44` has a pvalue of 0.67.

0.67*100% = 67%

67% of her laps that are completed in less than 130 seconds.

d. The fastest 3% of her laps are under

Under the 3rd percentile, which is X when Z has a pvalue of 0.03. So X when Z = -1.88.

`Z = (X - \mu)/(\sigma)`

`-1.88 = (X - 129.01)/(2.26)`

`X - 129.01 = -1.88*2.26`

`X = 124.8`

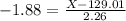
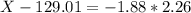
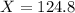
So the answer is 124.8 seconds.

e. The middle 80% of her laps are from ___________seconds to ________seconds.

The 50 - (80/2) = 10th percentile to the 50 + (80/2) = 90th percentile.

10th percentile:

X when Z has a pvalue of 0.1, so X when Z = -1.28.

`Z = (X - \mu)/(\sigma)`

`-1.28 = (X - 129.01)/(2.26)`

`X - 129.01 = -1.28*2.26`

`X = 126.1`

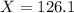
90th percentile:

X when Z has a pvalue of 0.9, so X when Z = 1.28.

`Z = (X - \mu)/(\sigma)`

`1.28 = (X - 129.01)/(2.26)`

`X - 129.01 = 1.28*2.26`

`X = 131.9`

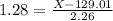
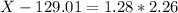
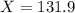
The middle 80% of her laps are from 126.1 seconds to 131.9 seconds.