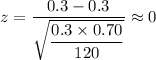Answer:
The test statistic for Business College is -0.9944
The test statistic for Liberal Arts College is 1.1483
The test statistic for Education College is 0
Explanation:
The percentage of ABC University college students in
Business College = 35%
Liberal Arts College = 35%
Education College = 30%
The number of students in the sample = 300 students
In the sample, the number of students in;
Business College = 90
Liberal Arts College = 120
Education College = 90
Therefore;
The percentage of Business College students = 90/300 × 100 = 30%
The percentage of Liberal Arts College, = 120/300 × 100 = 40%
The percentage of Education College,
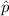 = 90/300 × 100 = 30%
= 90/300 × 100 = 30%
The test statistic for the difference in two proportions is given as follows;
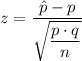
Where;
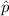 = The sample proportion
= The sample proportion
p = The population proportion
q = 1 - p
n = The sample size
The test statistic for Business College is;
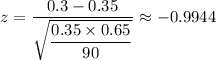
The test statistic for Liberal Arts College is;
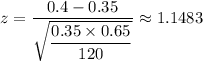
The test statistic for Education College is;