Question

Assume that the Poisson distribution applies and that the mean number of hurricanes in a certain area is 5.3 per year. a. Find the probability that in a year, there will be 3 hurricanes. b. in a 45-year period, how many years are expected to have 3 hurricanes ? c. How does the result from part (b) compare to a recent period of 45 years in which 5 years had 3 hurricanes? Does the Poisson distribution work well here?

Answer 1

a) 0.12386 = 12.386% probability that in a year, there will be 3 hurricanes.

b) 5.57 years are expected to have 3 hurricanes, that is, between 5 and 6 years.

c) 5 years is very close to the mean of 5.57, which means that the Poisson distribution works well here.

Explanation:

In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

In which

x is the number of sucesses

e = 2.71828 is the Euler number

`\mu` is the mean in the given interval.

The mean number of hurricanes in a certain area is 5.3 per year.

This means that
`\mu = 5.3`

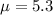
a. Find the probability that in a year, there will be 3 hurricanes.

This is P(X = 3).

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

`P(X = x) = (e^(-5.3)*5.3^(3))/((3)!) = 0.12386`

0.12386 = 12.386% probability that in a year, there will be 3 hurricanes.

b. in a 45-year period, how many years are expected to have 3 hurricanes ?

0.12386 each year. So, for 45 years:

45*0.12386 = 5.57

5.57 years are expected to have 3 hurricanes, that is, between 5 and 6 years.

c. How does the result from part (b) compare to a recent period of 45 years in which 5 years had 3 hurricanes? Does the Poisson distribution work well here?

5 years is very close to the mean of 5.57, which means that the Poisson distribution works well here.