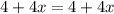Question

No Solutions 6−3+4x+1= x + One Solution 6−3+4x+1= x + Infinitely Many Solutions 6−3+4x+1= x +

Answer 1

Complete Question:

Complete each equation, so the statement about its solution is true.

No solutions:

6-3+4x+1= __x + __

One solution:

6-3+4x+1= __x + __

Infinitely many solutions:

6-3+4x+1 = __x + __

Answer:

`4 + 4x = 4x + 3` --- No solution

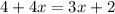`4 + 4x = 3x + 2` --- One solution

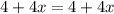`4 + 4x = 4 + 4x` --- Infinitely Many solution

Explanation:

Solving (a): No solutions

6-3+4x+1= __x + __

Collect like terms

6-3+1+4x= __x + __

4+4x= __x + __

To get no solution, the coefficient of x on both sides must be the same. However, the constant must be different.

So, we can equate the expression to:

`4 + 4x = 4x + 3`

Solving (b): One solution

6-3+4x+1= __x + __

This gives (same as (a))

4+4x= __x + __

To get one solution, the coefficient of x on both sides must be different. The constant can have any value.

So, we can equate the expression to:

`4 + 4x = 3x + 2`

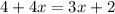
Solving (c): Infinitely many solutions

6-3+4x+1= __x + __

This gives (same as (a))

4+4x= __x + __

To get infinitely many solutions, both sides of the equation must be equal.

So:

`4 + 4x = 4 + 4x`