Question

A and C are the points (1, 3, −2) and (4, −4, 4) respectively. Point B divides AC in the ratio 1: 2. Find the coordinates of B.

Answer 1

Given:

The two points are A(1, 3, −2) and C(4, −4, 4).

Point B divides AC in the ratio 1: 2.

To find:

The coordinates of B.

Solution:

If a point divides a lines segment in m:n, then the coordinates of that point are:

`Point=\left((mx_2+nx_1)/(m+n),(my_2+ny_1)/(m+n),(mz_2+nz_1)/(m+n)\right)`

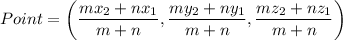
Point B divides AC in the ratio 1: 2. So, the coordinates of point B are:

`Point=\left((1(4)+2(1))/(1+2),(1(-4)+2(3))/(1+2),(1(4)+2(-2))/(1+2)\right)`

`Point=\left((4+2)/(3),(-4+6)/(3),(4-4)/(3)\right)`

`Point=\left((6)/(3),(2)/(3),(0)/(3)\right)`

`Point=\left(2,(2)/(3),0\right)`

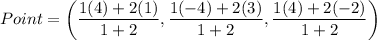
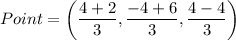
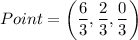
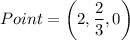
Therefore, the coordinates of B are
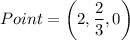`Point=\left(2,(2)/(3),0\right)`.