Given:
Total amount invested = $3000
Rate of annul interest on investments are 5% and 8%.
Total interest earned for the year = $210
To find:
The amount invested at each rate.
Solution:
Let the amount invested at 5% be
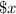 and the amount invested at 8% be
and the amount invested at 8% be
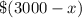 .
.
Total interest earned for the year = $210
It means. 5% of
 + 8% of
+ 8% of
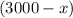 = 210
= 210
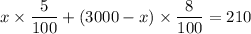
Multiply both sides by 100.
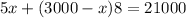
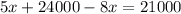
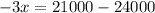
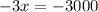
Divide both sides by -3.
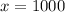
Now,

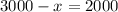
Therefore, the amount invested at the rate of 5% is $1000 and the amount invested at the rate of 8% is $2000.