Answer:
Time of murder = 10:39 am
Explanation:
Let the equation of exponential function representing the final temperature of the body after time 't' is,
f(t) =

Here, a = Initial temperature
n = Constant for the change in temperature
t = Duration
At 11:30 am temperature of the body was 91.8°F.
91.8 =
 --------(1)
--------(1)
Time to reach the body to the morgue = 12:30 pm
Duration to reach = 12:30 p.m. - 11:30 a.m.
= 1 hour
Therefore, equation will be,
84.4 =

eⁿ =
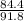
ln(eⁿ) = ln(0.9194)
n = -0.08403
From equation (1),
91.8 =
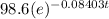
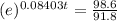
![ln[(e)^(0.08403t)]=ln[(98.6)/(91.8)]](https://img.qammunity.org/2022/formulas/mathematics/college/b6naxm19enay1mg9wf2u5ji37olcvs7e6y.png)
0.08403t = 0.07146
t = 0.85 hours
t ≈ 51 minutes
Therefore, murder was done 51 minutes before the detectives arrival.
Time of murder = 11:30 - 00:51
= 10:90 - 00:51
= 10:39 am