Answer:
m(∠EFG) = 69°.
Explanation:
Secants DF and HF intersect each other outside the circle at point F.
By the theorem,
"Measure of angle between intersecting secants is half of the difference of intercepted arcs"
m(∠EFG) =
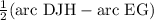
=
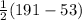
=
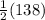
= 69°
Therefore, measure of angle EFG is 69°.