Answer:
The ball height is 11 meters at t = 0.59 seconds and t = 3.41 seconds.
Explanation:
Solving a quadratic equation:
Given a second order polynomial expressed by the following equation:
 .
.
This polynomial has roots
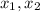 such that
such that
 , given by the following formulas:
, given by the following formulas:
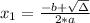

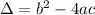
The height of the ball after t seconds is given by:
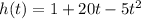
Find all values of t for which the ball's height is 11 meters.
This is t for which
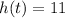 . So
. So
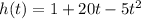
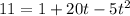
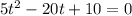
Simplifying by 5
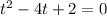
Which means that
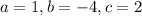

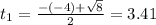

The ball height is 11 meters at t = 0.59 seconds and t = 3.41 seconds.