Question

Overbooking flights is a common practice of most airlines. A particular airline, believing that 5% of passengers fail to show for flights, overbooks (sells more tickets than there are seats). Suppose that for a particular flight involving a jumbo-jet with 265 seats, the airline sells 278 tickets. Question 1. What is the expected number of ticket holders that will fail to show for the flight

Answer 1

The expected number of ticket holders that will fail to show for the flight is 13.9.

Explanation:

For each ticket holder, there are only two possible outcomes. Either they fail to show up for the flight, or they do not. Ticket holders are independent. This means that we use the binomial probability distribution to solve this question.

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

The expected value of the binomial distribution is:

`E(X) = np`

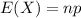
5% of passengers fail to show for flights

This means that
`p = 0.05`

278 tickets sold

This means that
`n = 278`

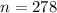
What is the expected number of ticket holders that will fail to show for the flight?

`E(X) = np = 278*0.05 = 13.9`

The expected number of ticket holders that will fail to show for the flight is 13.9.