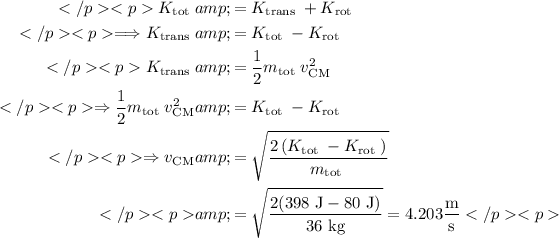
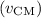Answer:
4.203 m/s
Step-by-step explanation:
First, we solve for the translational kinetic energy (
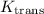 ) in the definition of the total kinetic energy
) in the definition of the total kinetic energy
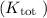 . We also have an equation for the definition of
. We also have an equation for the definition of
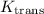 (third line). From these two equations we can solve for the speed of the center of mass
(third line). From these two equations we can solve for the speed of the center of mass