Answer:
The integral of the volume is:
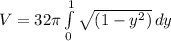
The result is:
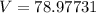
Explanation:
Given
Curve:
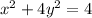
About line
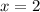 --- Missing information
--- Missing information
Required
Set up an integral for the volume
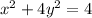
Make x^2 the subject

Square both sides

Factor out 4
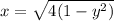
Split
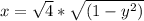
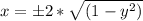
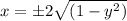
Split
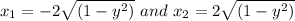
Rotate about x = 2 implies that:

So:
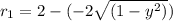

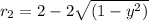
Using washer method along the y-axis i.e. integral from 0 to 1.
We have:
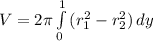
Substitute values for r1 and r2
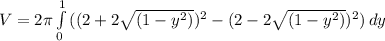
Evaluate the squares
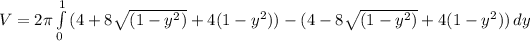
Remove brackets and collect like terms
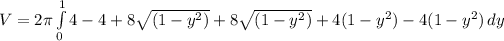
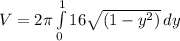
Rewrite as:
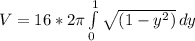
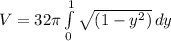
Using the calculator:

So:
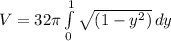
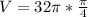
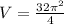
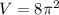
Take:

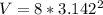
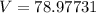 --- approximated
--- approximated