Question:
A system of equations is given below.
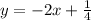 and
and
 .
.
What is true about both lines?
Answer:
They have the same slope but different y-intercepts, so they have no solution.
Explanation:
Given
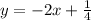

Required
Which is true?
A linear equation is represented as:

Where


For:
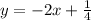
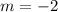
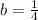
For

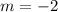
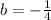
This implies that both equations have the same slope but different y intercept.
When two equations have the same slope but different y intercept; such system of equation has no solution.