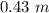Answer: 0.43 m
Step-by-step explanation:
Given
mass of bullet
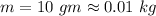
mass of block

The Force constant of spring is
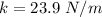
Speed of bullet is
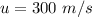
Conserving the energy i.e. kinetic energy of the bullet and box is converted into Elastic potential energy of spring

Conserving linear momentum
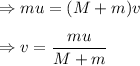
Put the value of
 we get
we get

Thus, spring will be compressed to a distance of