Question

A researcher wants to estimate the percentage of all adults that have used the Internet to seek pre-purchase information in the past 30 days, with an allowable sampling error (E) of 0.03 and a confidence level of 99.74%. Of secondary data indicated that 25% of all adults had used the Internet for such a purpose, what is the sample size

Answer 1

The sample size is 1875.

Explanation:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

In which

z is the zscore that has a pvalue of
`1 - (\alpha)/(2)`.

The margin of error is:

`M = z\sqrt{(\pi(1-\pi))/(n)}`

Sampling error of 0.03.

This means that
`M = 0.03`

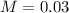
99.74% confidence level

So
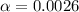`\alpha = 0.0026`, z is the value of Z that has a pvalue of
`1 - (0.0026)/(2) = 0.9987`, so
`Z = 3`.

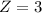25% of all adults had used the Internet for such a purpose

This means that
`\pi = 0.25`

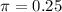
What is the sample size

The sample size is n. So

`M = z\sqrt{(\pi(1-\pi))/(n)}`

`0.03 = 3\sqrt{(0.25*0.75)/(n)}`

`0.03√(n) = 3√(0.25*0.75)`

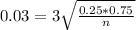
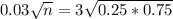
Simplifying by 0.03 both sides

`√(n) = 100√(0.25*0.75)`

`(√(n))^2 = (100√(0.25*0.75))^2`

`n = 1875`

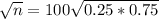
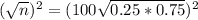
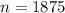
The sample size is 1875.