Question

A conducting coil of 1470 turns is connected to a galvanometer, and the total resistance of the circuit is 56.0 . The area of each turn is 4.32 x 10-4 m2. This coil is moved from a region where the magnetic field is zero into a region where it is nonzero, the normal to the coil being kept parallel to the magnetic field. The amount of charge that is induced to flow around the circuit is measured to be 9.18 x 10-3 C. Find the magnitude of the magnetic field. (Such a device can be used to measure the magnetic field strength and is called a flux meter).

Answer 1

B = - 1.51 10⁻⁷ T

Step-by-step explanation:

For this exercise we can use Faraday's law of induction

E = -
`- (d \phi _B)/(dt) = - (d (B A cos \theta ))/(dt)`

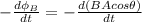
In this case, they indicate that the normal and the magnetite field are in the same direction, so the angle is zero (cos 0 = 1), they give the area of ​​the loop A = 4.32 10⁻⁴ m² and since we have N = 1470 turns in each one a voltage is induced

E = - N B A

B = -E A / N (1)

we find the induced voltage with ohm's law

V = i R

where the current is defined by

i = Q / t

we substitute

V = Q R / t

let's calculate

V = 9.18 10-3 56.0 / t

We must assume a time normally is t = 1 s

V = 0.514 V

this is the voltage in the circuit which must be the induced voltage V = E

we substitute in 1

B = - 0.514 4.32 10⁻⁴ / 1470

B = - 1.51 10⁻⁷ T