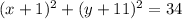Question

The diameter of a circle has endpoints (2,-6) and (-4,-16).

Enter an equation for the circle in standard form.

Answer 1

`(x + 1)^(2) + (y + 11)^(2) = 34`

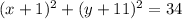
Explanation:

First find the midpoint of the segment from (2, -6) to (-4, -16)

((2 - 4)/2, (-6 - 16)/2)

(-2/2, -22/2)

(-1, -11) is the midpoint and is the center of the circle

Now find the distance between the 2 given points and divide by 2 to get the radius of the circle

d =
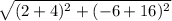
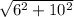
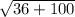
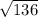
`\sqrt{(2 + 4)^(2) + (-6 + 16)^(2) }`

=
`\sqrt{6^(2) + 10^(2) }`

=
`√(36 + 100)`

=
`√(136)`

r =
`√(136)`/2

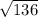`r^(2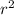)` = 136/4 = 34

Equation of circle with center at (-1, -11) and radius =
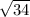`√(34)` is

`(x + 1)^(2) + (y + 11)^(2) = 34`