Question

In the diagram below DE is the midsegment of AABC.
Find the length of DE and BC.

Answer 1

DE = 5

BC = 10

Explanation:

ΔAED is a right triangle with legs of 4 and 6/2 = 3

Use the Pythagorean theorem to find DE

`4^(2) + 3^(2) = DE^(2)`

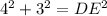
16 + 6 = 25 =
`DE^(2)`

DE = 5

The midsegment of a triangle is 1/2 the length of the corresponding side.

Therefore, BC = 2 DE = 2(5) = 10 = BC