Question

Aanswer (a) and (b)

a) a line pq is parallel to the line MN

b) The line MN is four times the length of the line pq​

Answer 1

Explanation:

a). From the given picture,

It's given: PM = 3(LP) and QN = 3(QN)

In ΔLPQ and ΔLMN,

LM = LP + PM

= LP + 3LP

= 4(LP)

Similarly, LN = 4(LQ)

By the converse property of similar triangles,

Corresponding sides of the triangles are proportional.

Therefore, their angles will be equal in measure.

∠LPQ ≅ ∠LMN and ∠LQP ≅ ∠LNM

Line PQ will be parallel to the line MN (By Converse of corresponding angle theorem).

b). Since, ΔLPQ ~ ΔLMN their corresponding sides will be in the same ratio.

`(LP)/(LM)=(LQ)/(LN)=(PQ)/(MN)`

`(LP)/(LM)=(PQ)/(MN)`

`(LP)/(4LP)=(PQ)/(MN)`

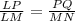
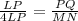
MN = 4PQ