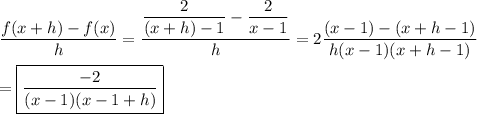Answer:
- (a) 40; (b) -8; (c) -5
- -2/((x -1)(x -1 -h))
Explanation:
1)
All of the values in parentheses are less than 6, so you only need the first part of the function definition.
f(x) = x^2 +6x = (x +6)x
f(4) = (4+6)(4) = 40
f(-2) = (-2 +6)(-2) = -8
f(-1) = (-1 +6)(-1) = -5
__
2)