Question

The points (-1,r) and (11, -1) lie on a line with slope 3/4
Find the missing coordinate r.

Answer 1

The value of the missing coordinate 'r' is -10

Explanation:

The two points have the following given parameters;

The point coordinates = (4, -17) and (8, r)

The line on which the two points rests has a slope of, m = 3/4

To find the value of 'r', we use the slope formula as follows;

`Slope, \, m =(y_(2)-y_(1))/(x_(2)-x_(1))`

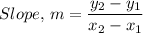
Plugging in the values gives;

`m = (3)/(4) = (-1-r)/(11-(-1)) =(-1-r)/(11 + 1) = (-1 - r)/(12)`

`(3)/(4) =(-1 - r)/(12)`

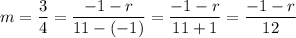
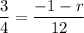
∴ 3 × 12 = 4 × (-1 - r)

36 = -4 - 4·r

36 + 4 = -4·r

r = 40/(-4) = -10

r = -10