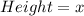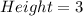Answer:
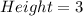
Explanation:
Given
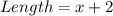
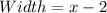
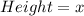
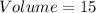
Required
Find the height
The volume is calculated as thus:
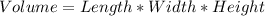
This gives
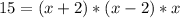
Apply difference of two squares
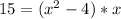
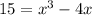
Subtract 15 from both sides
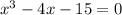
Using a calculator to factorize, we have:
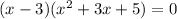
Split
 or
or
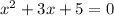
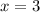 or
or
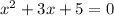
x has complex roots for
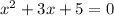
Hence:
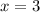
Recall that: