Given:
The rate of interest on three accounts are 7%, 8%, 9%.
She has twice as much money invested at 8% as she does in 7%.
She has three times as much at 9% as she has at 7%.
Total interest for the year is $150.
To find:
Amount invested on each rate.
Solution:
Let x be the amount invested at 7%. Then,
The amount invested at 8% = 2x
The amount invested at 9% = 3x
Total interest for the year is $150.
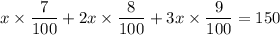
Multiply both sides by 100.
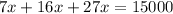

Divide both sides by 50.
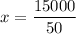
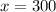
The amount invested at 7% is
 .
.
The amount invested at 8% is
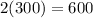
The amount invested at 9% is
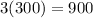
Thus, the stockbroker invested $300 at 7%, $600 at 8%, and $900 at 9%.