Answer:
40 feet (after 1.5 seconds).
Explanation:
The height h of the ball after t seconds is modeled by the function:
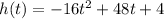
And we want to determine the ball's maximum height.
Since the given function is a quadratic, the maximum height occurs at the vertex point.
For quadratics, the vertex point is given by the formulas:
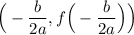
In this case, a = -16, b = 48, and c = 4.
Therefore, the t-coordinate at which the vertex occurs is:

So, the maximum height occurs after 1.5 seconds.
Then the maximum height will be:

So, the maximum of the ball is 40 feet (after 1.5 seconds).