Given:
The function is:
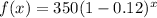
To find:
The initial value of the function.
Solution:
We have,
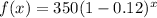
The value of the function at x=0 is called the initial value of the function.
For x=0, we get
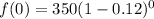
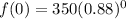
Clearly 0.88 is a non zero number and zero to the power of a non zero number is always 1.
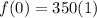
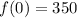
Therefore, the initial value of the function is 350.