Question

If my angular velocity slows from 30 Hz to 20 Hz in 72°, what is my deceleration?

Answer 1

The deceleration is -7.85x10³ rad/s².

Step-by-step explanation:

The angular speed (ω) is related to frequency (f) as follows:

`\omega = 2\pi f`

When the frequency is 30 Hz the angular speed is:

`\omega_(i) = 2\pi f = 2\pi*30 Hz = 188.5 rad/s`

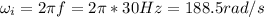
Now, when the frequency is 20 Hz the angular speed is:

`\omega_(f) = 2\pi*20 Hz = 125.7 rad/s`

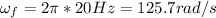
Finally, the angular acceleration (α) can be found using the following equation:

`\omega_(f)^(2) = \omega_(i)^(2) + 2\theta \alpha`

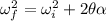
Where:

θ: is the angular displacement = 72°

`\alpha = (\omega_(f)^(2) - \omega_(i)^(2))/(2\theta)`

`\alpha = ((125.7 rad/s)^(2) - (188.5 rad/s)^(2))/(2*72*(2\pi rad)/(360)) = -7.85 \cdot 10^(3) rad/s^(2)`

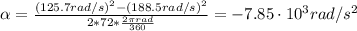
Therefore, the deceleration is -7.85x10³ rad/s².

I hope it helps you!