Question

State the common factor of the terms in the polynomial 5x^2 + 30x^2 - 10x. Then factor the polynomial

Answer 1

The common factor is 5x.

The factored polynomial is:
`5x(7x - 2)`

Explanation:

Factor of the numbers:

The common factor of the numbers 5, 30 and 10 is 5, as it is the greater common divisor of them, as found below:

5 - 30 - 10|5

1 - 6 - 2

Factor of the exponents:

Between
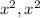`x^2, x^2` and
`x`, the common factor is the x with the lowest exponent. So x

Common factor

Number multiplied by exponents, so 5x.

Factoring the polynomial:

`5x^2 + 30x^2 - 10x = 5x((5x^2)/(5x) + (30x^2)/(5x) - (10x)/(5x)) = 5x(x + 6x - 2) = 5x(7x - 2)`

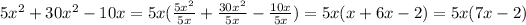
The factored polynomial is:
`5x(7x - 2)`