Question

Compare and order the numbers from least to greatest by dragging them to the boxes. Plot the numbers on the number line to help.

Answer 1

The first one should be 6.8 I hope you get it right

Answer 2

Ordered from least to greatest, the numbers are:

`\( (46)/(7) \) (6.57), 6.6, 6.8, \( √(47) \) (6.86)`.

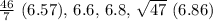To compare and order the numbers from least to greatest, follow these steps:

1. Simplify the Square Root: First, we need to simplify the square root of 47. Since 47 is not a perfect square, we'll need to find the two perfect squares it lies between. We know that
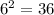`\( 6^2 = 36 \)` and
`\( 7^2 = 49 \)`. So,
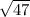`\( √(47) \)` is between 6 and 7, but closer to 7 because 47 is closer to 49.

2. Divide 46 by 7: Next, we divide 46 by 7 to get an exact decimal. This will help us to compare it with the other decimals.

3. List the Numbers: The numbers given are 6.6,
`\( √(47) \)`,
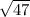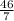`\( (46)/(7) \)`, and 6.8.

4. Compare and Order: We need to compare these numbers to place them in order from least to greatest.

Let's perform the calculations for steps 1 and 2.

The square root of 47 is approximately 6.86, and 46 divided by 7 is approximately 6.57.

Now we have all the numbers in decimal form:

• 6.6
• 
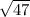  `\( √(47) \)` ≈ 6.86
• 
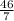  `\( (46)/(7) \)` ≈ 6.57
• 6.8

To plot these numbers on the number line, you would place:

• 
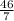  `\( (46)/(7) \)` (6.57) just a little past the midpoint between 6.5 and 6.6.
• 6.6 directly above the corresponding point on the number line.
• 6.8 directly above the corresponding point on the number line.
• 
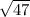  `\( √(47) \)` (6.86) just a little before 6.9 on the number line.

The exact positions would depend on the scale of the number line, but these approximations should help you visualize their placement.