Answer:
Follows are the solution to this question:
Explanation:
Given:
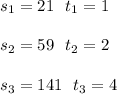
All points are on the graph including its quadratic function, in order to install their coordinates to values of x and y with the following values:
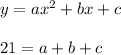 Inserting the coordinates of the first point
Inserting the coordinates of the first point
 Inserting the coordinates of the second point
Inserting the coordinates of the second point
 Inserting the coordinates of the third point
Inserting the coordinates of the third point