Question

A flat loop of wire consisting of a single turn of cross-sectional area 8.80 cm2 is perpendicular to a magnetic field that increases uniformly in magnitude from 0.500 T to 1.80 T in 1.10 s. What is the resulting induced current if the loop has a resistance of 2.20

Answer 1

The magnitude of the induced current is 4.73 x 10⁻³ A.

Step-by-step explanation:

Given;

number of turns, N = 1

cross sectional area of the loop, A = 8.8 cm² = 8.8 x 10⁻⁴ m²

change in magnetic field strength, ΔB = 1.8 T - 0.5 T = 1.3 T

change in time, Δt = 1.10 s

resistance of the loop, R = 2.2 ohm

The magnitude of the induced emf is calculated as;

`emf = (NA \Delta B)/(\Delta t) \\\\emf = (1 * 8.8* 10^(-4) * 1.3)/(1.10) \\\\emf = 1.04 * 10^(-3) \ V`

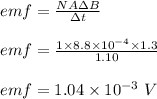
The induced current in the loop is calculated as;

`I = (emf)/(R) \\\\I = (1.04 * 10^(-3))/(2.2) \\\\I= 4.73 * 10^(-4) \ A`

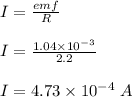
Therefore, the magnitude of the induced current is 4.73 x 10⁻³ A