Question

Please help me find the final answer

Answer 1

5. The mass of Na₂CO₃, that will produce 5 g of CO₂ is approximately 12.04 grams of Na₂CO₃

6. The mass of nitrogen gas (N₂) that will react completely with 150 g of hydrogen (H₂) in the production of NH₃ is 693.
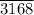`\overline{3168}` grams of N₂

Step-by-step explanation:

5. The given equation for the formation of carbon dioxide (CO₂) from sodium bicarbonate (Na₂CO₃) is presented as follows;

(Na₂CO₃) + 2HCl → 2NaCl + CO₂ + H₂O

One mole (105.99 g) of Na₂CO₃ produces 1 mole (44.01 g) of CO₂

The mass, 'x' g of Na₂CO₃, that will produce 5 g of CO₂ is given by the law of definite proportions as follows;

`(x \ g)/(105.99 \ g) = (5 \ g)/(44.01 \ g)`

`\therefore {x \ g} = (5 \ g)/(44.01 \ g) * 105.99 \ g \approx 12.04 \ g`

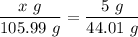
The mass of Na₂CO₃, that will produce 5 g of CO₂, x ≈ 12.04 g

6. The chemical equation for the reaction is presented as follows;

N₂ + 3H₂ → 2NH₃

Therefore, one mole (28.01 g) of nitrogen gas, (N₂), reacts with three moles (3 × 2.02 g) of hydrogen gas (H₂) to produce 2 moles of ammonia (NH₃)

The mass 'x' grams of nitrogen gas (N₂) that will react completely with150 g of hydrogen (H₂) in the production of NH₃ is given as follows;

`(x \ g)/(28 .01 \ g) = (150 \ g)/(3 * 2.02 \ g) = (150 \ g)/(6.06 \ g)`

`\therefore \ x \ g= (150 \ g)/(6.06 \ g) * 28.01 \ g = 693.\overline {3168} \ g`

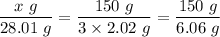
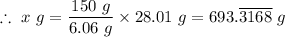
The mass of nitrogen gas (N₂) that will react completely with 150 g of hydrogen (H₂) in the production of NH₃, x = 693.
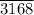`\overline{3168}` grams