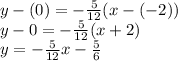Answer (assuming it can be in slope-intercept form):
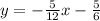
Explanation:
1) First, find the slope of the line between the two points by using the slope formula,
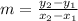 . Substitute the x and y values of the given points into the formula and solve:
. Substitute the x and y values of the given points into the formula and solve:
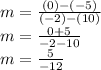
Thus, the slope of the line is
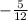 .
.
2) Next, use the point-slope formula
 to write the equation of the line in point-slope form. Substitute values for
to write the equation of the line in point-slope form. Substitute values for
 ,
,
 , and
, and
 in the formula.
in the formula.
Since
 represents the slope, substitute
represents the slope, substitute
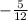 in its place. Since
in its place. Since
 and
and
 represent the x and y values of one point the line intersects, choose any of the given points (it doesn't matter which one, it will equal the same thing) and substitute its x and y values into the formula as well. (I chose (-2,0), as seen below.) Then, isolate y and expand the right side in the resulting equation to find the equation of the line in slope-intercept form:
represent the x and y values of one point the line intersects, choose any of the given points (it doesn't matter which one, it will equal the same thing) and substitute its x and y values into the formula as well. (I chose (-2,0), as seen below.) Then, isolate y and expand the right side in the resulting equation to find the equation of the line in slope-intercept form: