Question

Line m passes through point (-2,-1) and is perpendicular to the graph of y=-2/3x+6. Line n is parallel to line m and passes through the point (4,-3). What is the equation in slope-intercept form of line n?

Answer 1

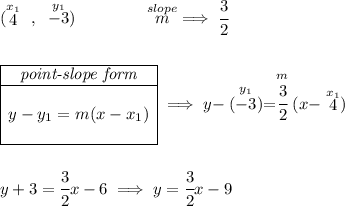
keeping in mind that perpendicular lines have negative reciprocal slopes, let's check for the slope of the equation above

`y=\stackrel{\stackrel{m}{\downarrow }}{-\cfrac{2}{3}} x+6\qquad \impliedby \begin{array} \cline{1-1} slope-intercept~form\\ \cline{1-1} \\ y=\underset{y-intercept}{\stackrel{slope\qquad }{\stackrel{\downarrow }{m}x+\underset{\uparrow }{b}}} \\\\ \cline{1-1} \end{array}`

well, we know that "m" is perpendicular to the line above, so that means that line "m" has a slope of

`\stackrel{~\hspace{5em}\textit{perpendicular lines have \underline{negative reciprocal} slopes}~\hspace{5em}} {\stackrel{slope}{-\cfrac{2}{3}} ~\hfill \stackrel{reciprocal}{-\cfrac{3}{2}} ~\hfill \stackrel{negative~reciprocal}{-\cfrac{3}{-2}\implies \cfrac{3}{2}}}`

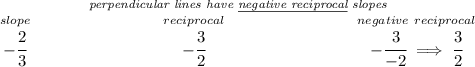
keeping in mind that parallel lines have exactly the same slope, and since we know that "n" is parallel with "m", that means that if "m" has a slope of 3/2 than "n" must have the same slope.

so we're really looking for the equation of a line whose slope is 3/2 and passes through (4 , -3)

`(\stackrel{x_1}{4}~,~\stackrel{y_1}{-3})\qquad \qquad \stackrel{slope}{m}\implies \cfrac{3}{2} \\\\\\ \begin{array}ll \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{(-3)}=\stackrel{m}{\cfrac{3}{2}}(x-\stackrel{x_1}{4}) \\\\\\ y+3=\cfrac{3}{2}x-6\implies y=\cfrac{3}{2}x-9`