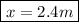Hi there!
1)
Since the charge placed in the middle is positive, we know that the particle is being repelled.
The particle experiences a greater repelling force by the 9μC charge. We can use the equation for electric force:
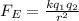
k = Coulomb's Constant
q₁, q₂: Charges (C)
r = distance between charges (m)
This is a VECTOR quantity, so we must subtract the forces since they point in opposite directions.
Force from 9μC particle:
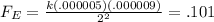
Force from 4μC particle:
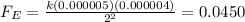
Subtract:
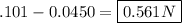
2)
We can find a position by setting the two equations equal to one another. (Both repelling forces must be EQUAL for the force = 0 N)
Let the distance between the 9μC and 5μC charge equal 'x', and the distance between the 4μC and 5μC charge equal '4 - x'.
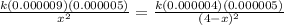
Cancel out 'k' and the 5μC value.
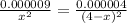
Solve for 'x' using a graphing utility.