Question

Seven times the opposite of a number is 50 less than 3 times the number. What is the number?

Answer 1

Answer: The number is 5

Creating an Equation:

First, we will need to write a mathematical equation that represents the problem.

-> If n is our number, then the opposite of n is -n because -1 times -n is n and -1 times n is -n

-> The underlined portion becomes a part of our equation in the next line.

Seven times the opposite of a number is 50 less than 3 times the number

7 * -n is 50 less than 3n

7 * -n is 3n - 50

7(-n) = 3n - 50

Solving for the Number:

7(-n) = 3n - 50 =|= Our equation

-7n = 3n - 50 =|= Distribute the 7 into -n

-10n = -50 =|= Subtract 3n from both sides of the equation

n = 5 =|= Divide both sides of the equation by -10

Our number is 5

Answer 2

Question :-

• Seven times the opposite of a number is 50 less than 3 times the number. What is the number?

Step-by-step explanation :-

• Let the number be " x. "

According to the question, it's given –

Seven times the opposite of a number is 50 less than 3 times the number.

We've assumed that the number is x. So, opposite of the number will be -x.

`\qquad`
`\pink{ \bf \longrightarrow 7(-x) = 3x-50}`

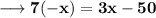
Now, let's solve this equation –

`\qquad`
`\bf \longrightarrow 7(-x) = 3x-50`

`\qquad`
`\sf \longrightarrow -7x = 3x -50`

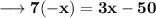
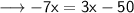
Subtract 3x from both side –

`\qquad`
`\sf \longrightarrow -7x -3x = 3x -50-3x`

Cancel 3x from right side –

`\qquad`
`\sf \longrightarrow -7x -3x =\cancel{ 3x} -50-\cancel{3x }`

`\qquad`
`\sf \longrightarrow -10x = -50`

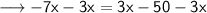
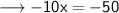
Now, cancel (-) sign from both side –

`\qquad`
`\sf \longrightarrow \cancel{-}10x = \cancel{-}50`

`\qquad`
`\sf \longrightarrow 10 x = 50`

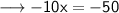
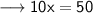
Then, divide both sides by 10 –

`\qquad`
`\sf \longrightarrow (10x)/(10) = (50)/(10)`

`\qquad`
`\sf \longrightarrow \frac{\cancel{10}x}{\cancel{10}} = \cancel{(50)/(10)}`

`\qquad`
`\pink{ \bf \longrightarrow x = 5 }`

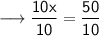
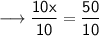
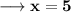
• Henceforth, the number will be 5.