Question

A radioactive isotope is decaying at a rate of 15% every hour. Currently there are 150 grams of the substance. Write an equation that will represent the number of grams present after n hours. How much will be left one day from now?A radioactive isotope is decaying at a rate of 15% every hour. Currently there are 150 grams of the substance. Write an equation that will represent the number of grams present after n hours. How much will be left one day from now?

Answer 1

The equation that will represent the number of grams present after n hours is
`A(n) = 150(0.85)^n`

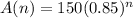
3.035 grams will be left one day from now.

Explanation:

Exponential equation for the amount of a substance:

The exponential equation for the amount of a substance is given by:

`A(t) = A(0)(1-r)^t`

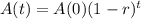
In which A(0) is the initial amount and r is the decay rate, as a decimal, and t is the time period.

A radioactive isotope is decaying at a rate of 15% every hour.

This means that
`r = 0.15`

Currently there are 150 grams of the substance.

This means that
`A(0) = 150`

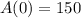
Write an equation that will represent the number of grams present after n hours.

`A(n) = A(0)(1-r)^n`

`A(n) = 150(1-0.15)^n`

`A(n) = 150(0.85)^n`

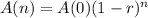
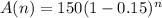
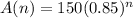
How much will be left one day from now?

One day is 24 hours, so this is A(24).

`A(24) = 150(0.85)^(24) = 3.035`

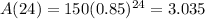
3.035 grams will be left one day from now.