Question

To compare two products such as colas, a random sample is selected to taste two types of colas (A and B). Each selected subject tastes both colas and selects his/her preferred cola. What sample size would be needed to ensure that the margin of error in the brand preference is less than 5 percentage points in more than 95% of experiments

Answer 1

Answer: The sample size would be needed = 385

Explanation:

Let p be the prior population proportion.

Margin of error = E

When not estimate of p is given , the formula to calculate the minimum sample size n =
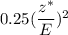`0.25((z^*)/(E))^2` , where z* = critical value for given confidence interval.

Here z* for 95% confidence level is 1.96.

E=5%=0.05

Then
`n=0.25((1.96)/(0.05))^2\approx385`

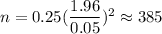
Hence, the sample size would be needed = 385