Answer:
The 99% confidence interval for the fraction of US adult Twitter users who get some news on Twitter is (0.4872, 0.6018). It means that we are 99% sure that the true proportion of US adult Twitter users who get some news on Twitter is between 0.4872 and 0.6018.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.
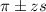
In which
z is the zscore that has a pvalue of
 , and s is the standard error.
, and s is the standard error.
54% of US adult Twitter users get at least some news on Twitter.
This means that
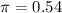
The standard error for this estimate was 2.4%
This means that

99% confidence level
So
 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
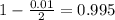 , so
, so
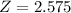
The lower bound is:
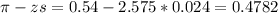
The upper bound is:
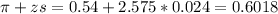
The 99% confidence interval for the fraction of US adult Twitter users who get some news on Twitter is (0.4872, 0.6018). It means that we are 99% sure that the true proportion of US adult Twitter users who get some news on Twitter is between 0.4872 and 0.6018.